Prof. Z. Etienne

Translations

April 2022 Update

After a year spent rewriting the software behind BlackHoles@Home, we're seeing beautiful results. In short, the software seems to do what it needs to do, but I'm still running it through some trials.

Continue to stay tuned to this website, but as results from trial simulations pour in, I'll be sharing results/updates on the below Twitter feed:

October 2021 Update

BlackHoles@Home is now hiring! We are looking for a postdoctoral researcher. Click here for more details, and click here to apply!

Also we've made great progress over the past few months, with testing of a new, far more flexible infrastructure for BlackHoles@Home underway. This infrastructure will enable not only the (complex) grid structures that work extremely well for the long inspiral phase (as shown below), but also the (simple) grid structures needed for the merger phase of the black holes (i.e., when two black holes coalesce to a single black hole). Also many improvements to its mother code NRPy+ (GitHub) have been imported to BlackHoles@Home, and vice versa.

July 2021 Update

BlackHoles@Home is moving to the University of Idaho. Stay tuned for a launch in Fall 2021!

April 2021 Update

TL;DR: Tremendous progress has been made!

Here is a technical update showing some of our latest results. Please refer to sections below this one for a more basic introduction to the project.

BlackHoles@Home is a proposed BOINC project that aims to fit binary black hole simulations on consumer-grade desktop computers. In doing so we can enlist the general public to help generate the large gravitational waveform catalogs that form the foundation for a great deal of gravitational wave science.

Traditionally, these binary black hole simulations have been performed on supercomputers. BlackHoles@Home implements new approaches for robustly solving Einstein's equations of general relativity in highly efficient coordinate systems, so that these simulations will fit on consumer-grade desktop computers in only a few gigabytes of RAM.

In particular BlackHoles@Home makes use of the following numerical grid structure:

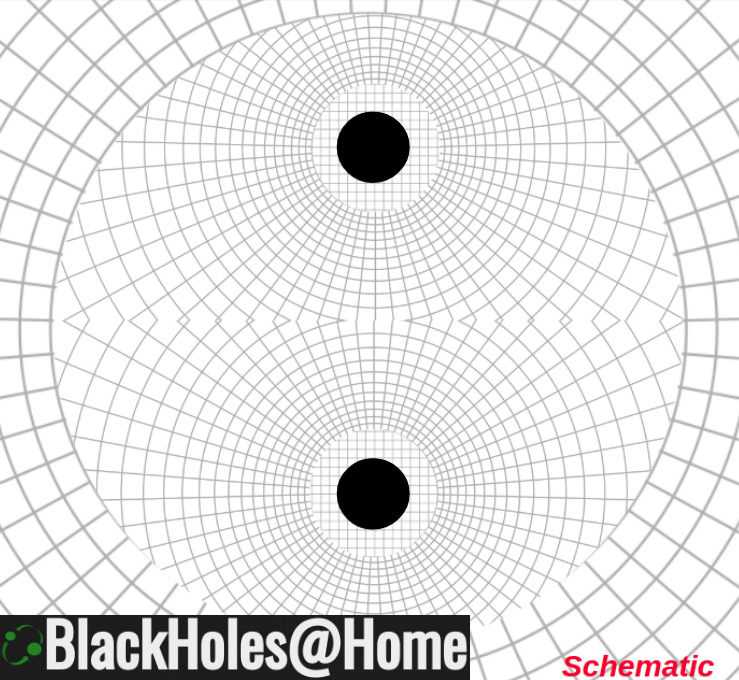
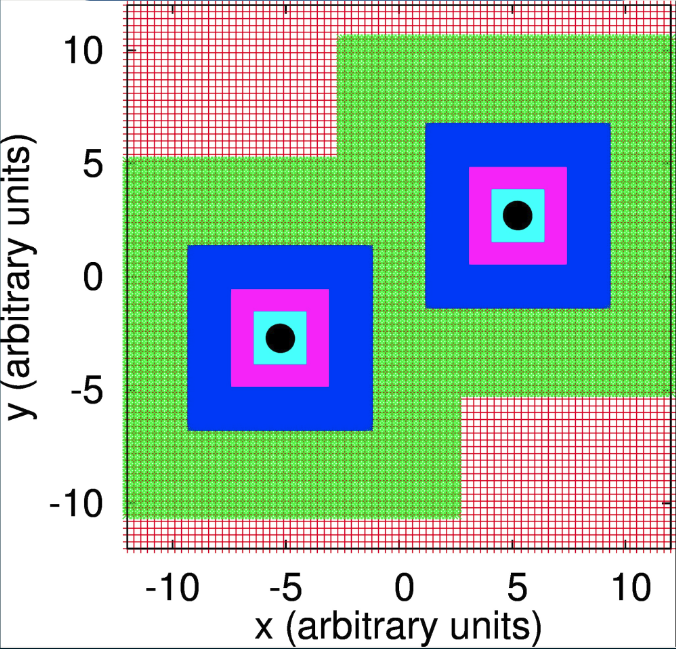
Compare this to the most popular approach for solving Einstein's equations, which does not exploit near-symmetries in the problem, instead opting for equally robust nested Cartesian grids at varying resolutions (adaptive mesh refinement):

We performed a simulation of the first black hole collision ever observed by gravitational wave detectors, which contributed to the 2017 Nobel Prize in Physics. The simulation was performed both using a trusted code (the Einstein Toolkit, which uses adaptive mesh refinement), and BlackHoles@Home. This happens to be an Einstein Toolkit gallery example, which provides an interesting and very well documented example of the Toolkit's capabilities. Also the ET simulation results are publicly available on Zenodo.

Basic initial properties of this binary black hole system:

  • 10M initial separation
  • q=36/29=1.24 mass ratio
  • 0.31 = spin component of more massive black hole (direction parallel to the orbital angular momentum vector)
  • -0.46 = spin component of less massive black hole (direction parallel to the orbital angular momentum vector)

Numerical details:

  • Both codes solve Einstein's equations using the BSSN formalism
  • Both codes compute partial derivatives with finite differences at 8th order accuracy
  • The ET simulation enables Kreiss-Oliger dissipation at 9th order, spatially constant with strength parameter set to 0.1.
  • BlackHoles@Home enables Kreiss-Oliger dissipation at 9th order, spatially varying such that it is effectively disabled inside the black holes and maximized in the weak-field region.
  • The ET numerical grids are as follows:
    • Strong-field region: Cartesian AMR grids, courtesy the Carpet code.
    • Weak-field region: Cubed sphere grids, courtesy the Llama code
    • Highest resolution of the Cartesian grids (near the black holes) is around M/52.
    • The outer boundary of the ET domain is 2200M.
  • BlackHoles@Home grids are as follows:
    • Strong-field region: Each black hole is housed within a small SinhCartesian AMR grid patch with one level of refinement; surrounded by a SinhSpherical grid. The SinhSpherical grids overlap.
    • The gravitational wavezone adopts a single SinhSpherical grid.
    • Highest resolution of the SinhCartesian grids (near the black holes) is around M/80.
    • The outer boundary of the BlackHoles@Home domain is 768M.

The BlackHoles@Home simulations in the following few plots use only 1.78GB of RAM, where the Einstein Toolkit uses 98GB.

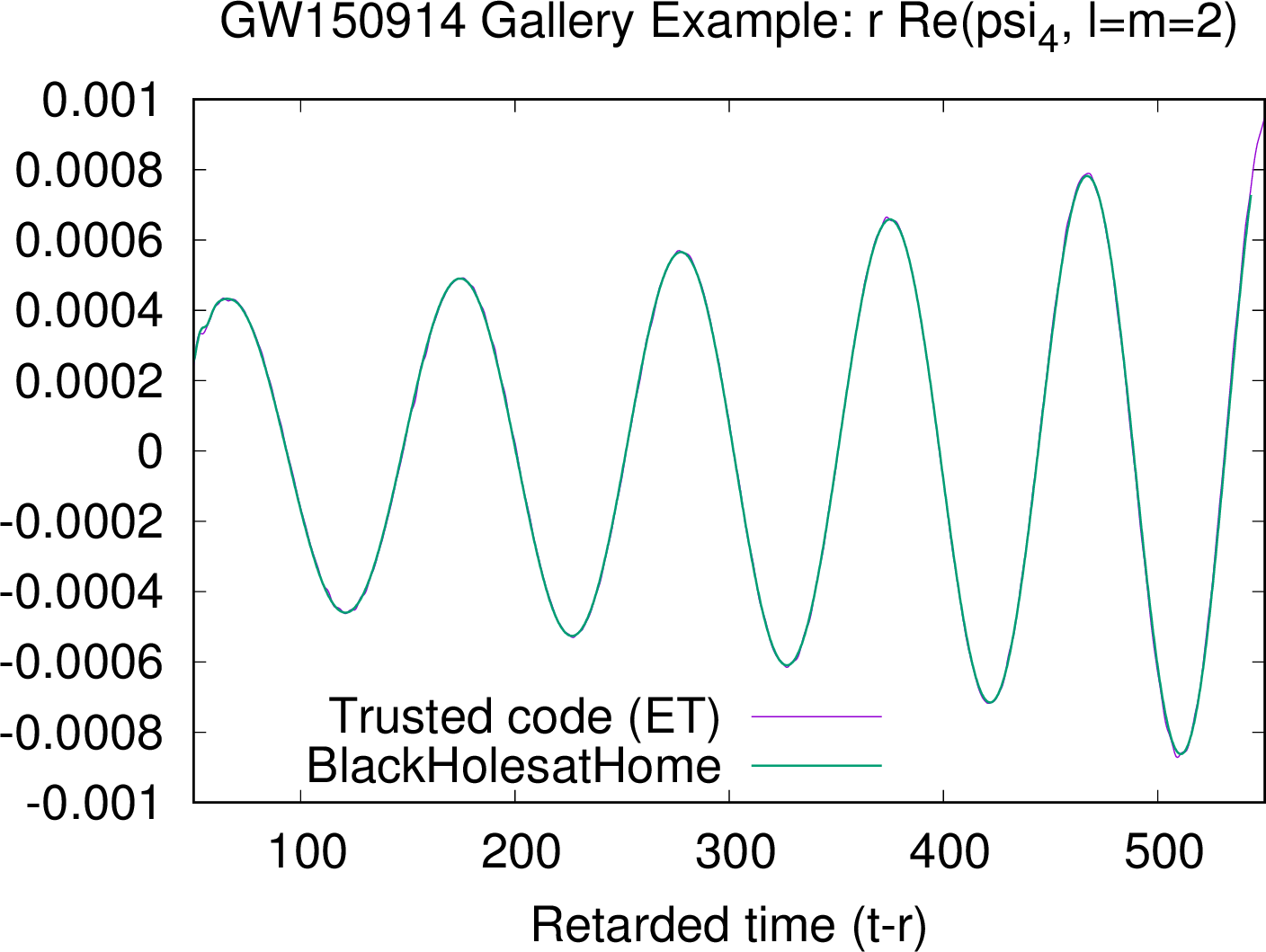
The payload of the simulation is a gravitational wave prediction. As is standard, we decompose the gravitational wave content into s=2 spin-weighted spherical harmonics for efficiency. The dominant (l=m=2) mode of gravitational waves (real part of psi_4; the second time derivative of gravitational-wave strain, extracted at r=100M in the ET and 108M in BlackHoles@Home) agrees extremely well with the trusted code:

Excitingly the dominant higher-order (l=m=4) mode of gravitational waves is not as noisy in BlackHoles@Home, as compared to the ET!

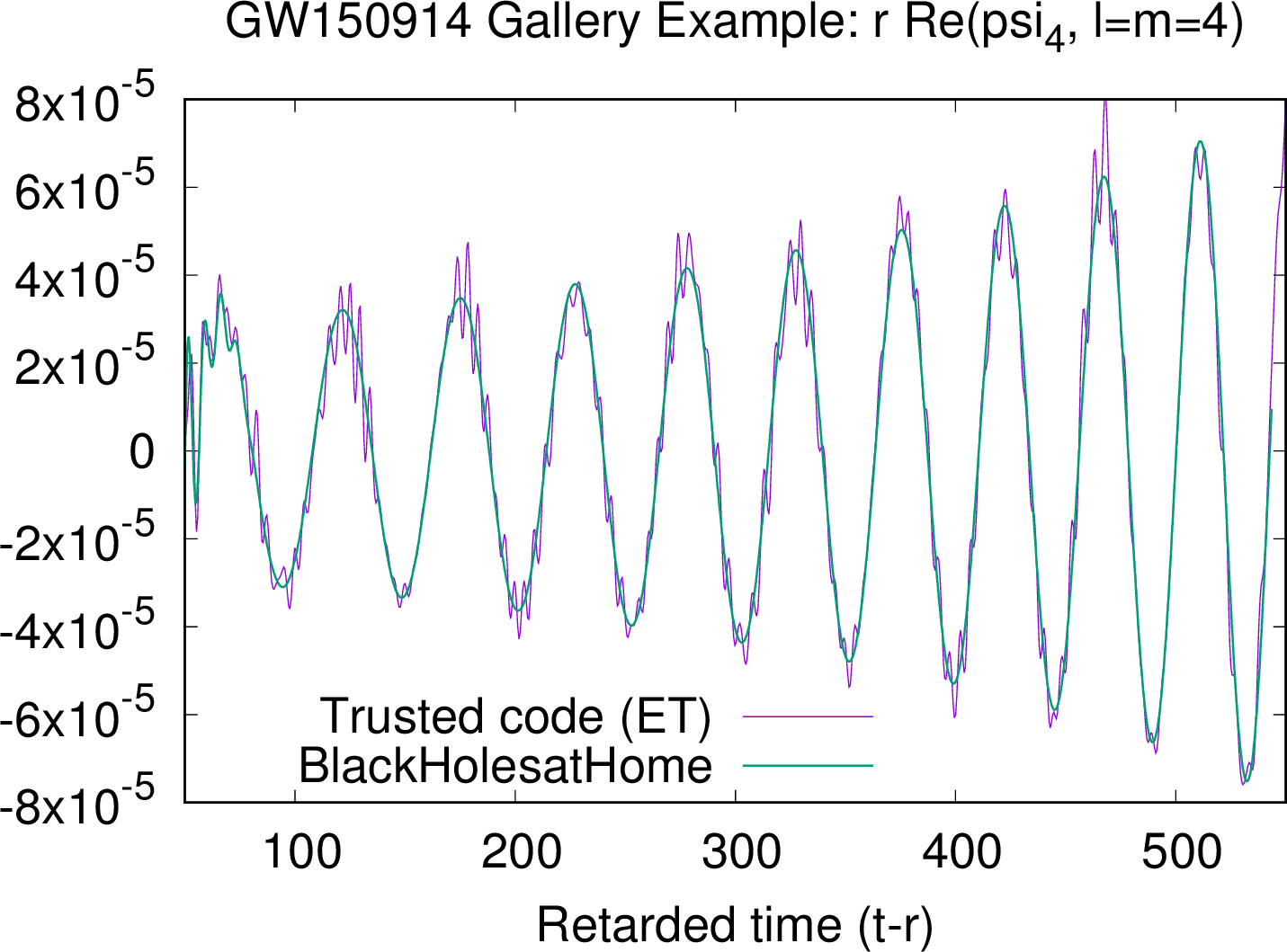
This noise stems from numerical errors as high-frequency waves reflect off of adaptive mesh refinement boundaries in space and time (see this paper, and this paper). The number of grid boundaries is minimized in the BlackHoles@Home grids, and as a result the numerical noise is much less.

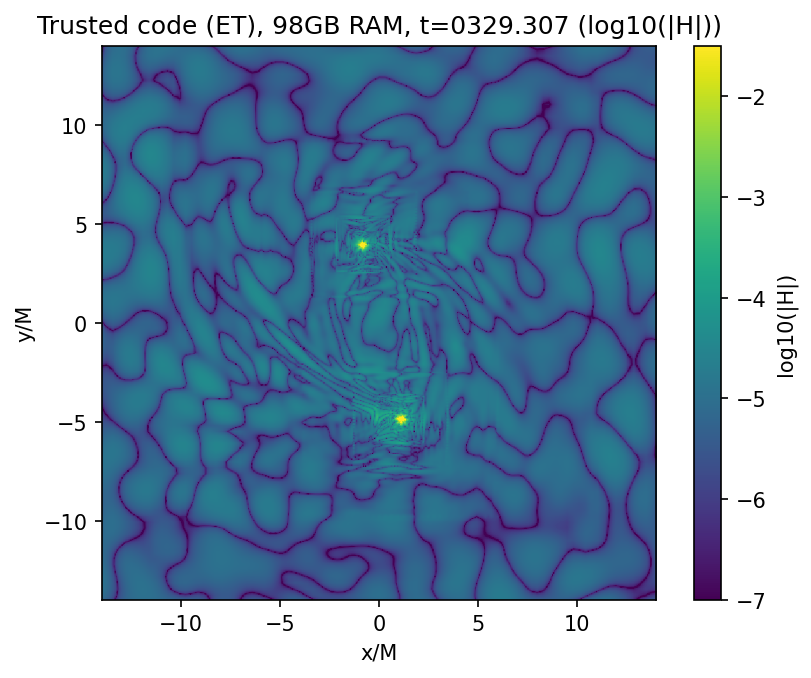
Next we show a measure of logarithmic numerical error (log10 absolute value of Hamiltonian constraint violation) on the orbital plane, after about 1.5 orbits of the binary black hole. The largest errors exist inside the black holes, thus the black holes appear as yellow dots.

First the result from the Einstein Toolkit (a trusted code), using 98GB of RAM:

Next from BlackHoles@Home, using only 1.78GB of RAM:

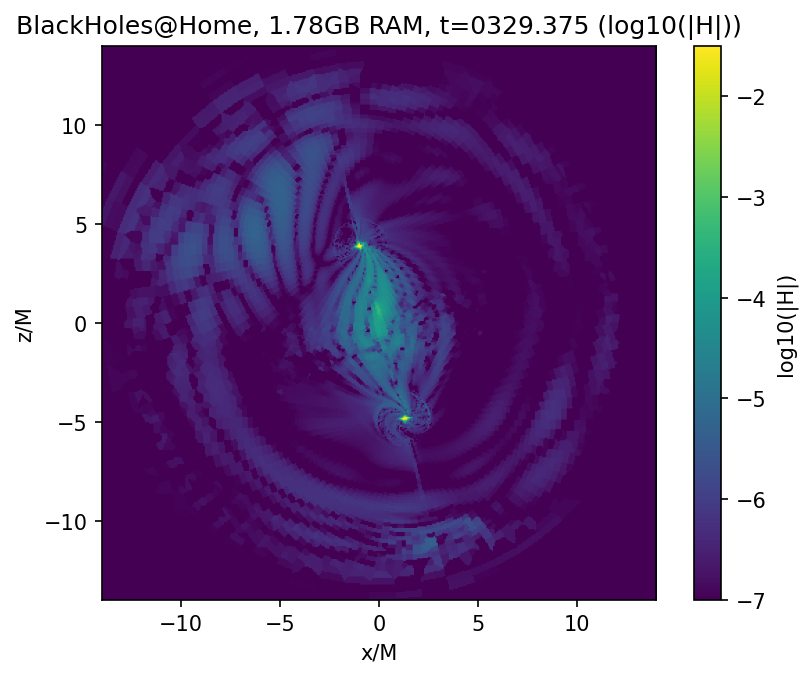
Notice the numerical errors are significantly smaller almost everywhere in BlackHoles@Home, as compared to the ET!

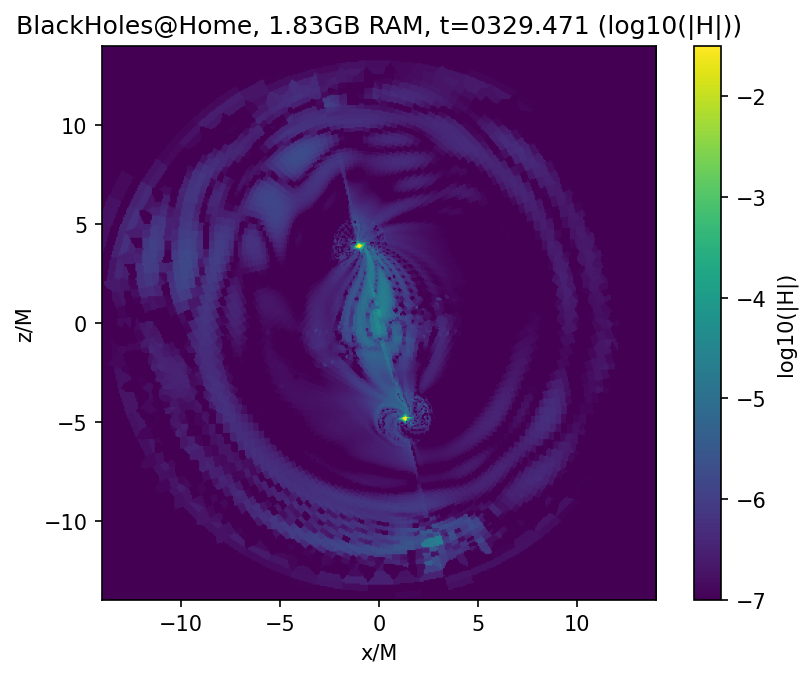
Upon further analysis we find the numerical errors are dominated by the small number of gridpoints sampling the polar angle in the small spherical grids surrounding each black hole. When this number is increased from 48 to 56, the errors drop significantly, and the memory usage increases only slightly to 1.83GB:

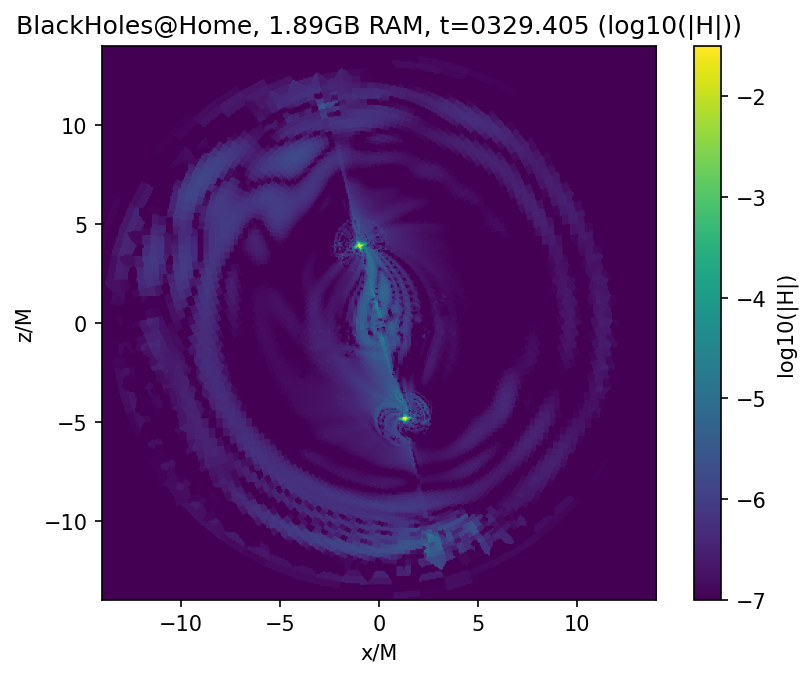
Increasing this to 64, the errors drop further (memory usage increases to 1.89GB):

Newsletter

Want to know what's on the horizon? Sign up for the BlackHoles@Home newsletter

Basic Introduction

Slides from the BlackHoles@Home APS 2019 press conference

Technical Introduction

When a gravitational wave is observed, answering the question "What exactly produced this?" is crucial to advancing science. Inferring physical properties of even the simplest observed gravitational wave source-black hole binaries-requires catalogs of numerical relativity gravitational waveforms spanning all seven dimensions of intrinsic parameter space (i.e., mass ratio, plus the three spin vector components of each black hole). Due to the requirement that virtually all numerical relativity simulations of black hole binaries to date be run on supercomputers, all such catalogs combined sample this parameter space to only about 3 points per dimension.

These tiny catalogs have been sufficient for noisy gravitational wave observations to date, as the noise acts to obscure the relatively small effects of misaligned spins, but they will not be good enough moving forward.

Colliding black holes!
Collide them yourself & learn how it's done at the Interactive NRPy+ Tutorial!

BlackHoles@Home aims to reduce the cost in memory of numerical relativity black hole and neutron star binary simulations by ~100x, through adoption of numerical grids that fully exploit near-symmetries in these systems. With this cost savings, black hole binary merger simulations can be performed entirely on a consumer-grade desktop (or laptop) computer.

BlackHoles@Home is destined to run on the BOINC infrastructure (alongside many other great "@home" projects), enabling anyone with a computer to contribute to construction of the largest numerical relativity gravitational wave catalogs ever produced.

Want to know what's on the horizon? Sign up for the BlackHoles@Home newsletter.

People

Zachariah B. Etienne is an associate professor of Physics & Astronomy at West Virginia University. Ian Ruchlin was a postdoctoral fellow at West Virginia University.

Back to top